
学术会议
【2023.11.17-11.17 北京】量化风险管理青年论坛
发布时间:2023-11-16
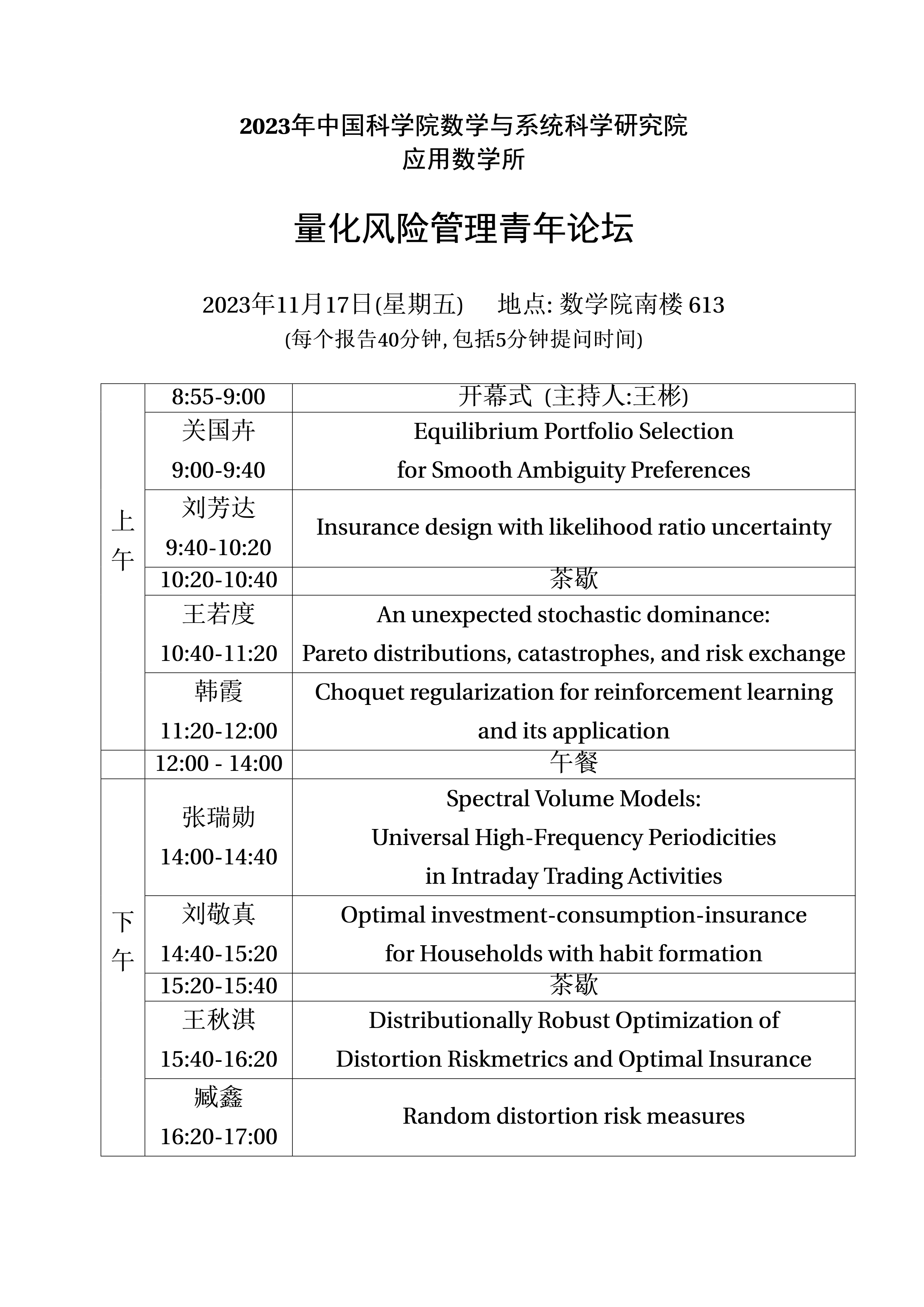
关国卉 (中国人民大学)
题目:Equilibrium Portfolio Selection for Smooth Ambiguity Preferences
摘要: This paper investigates the equilibrium portfolio selection for smooth ambiguity preferences in a continuous time market. The investor is uncertain about the risky asset’s drift term and updates the subjective belief according to the Bayesian rule. Two versions of the verification theorem are established and an equilibrium strategy can be decomposed into a myopic demand and two hedging demands. When the prior is Gaussian, the closed-form equilibrium solution is obtained. A puzzle in the numerical results is interpreted via an alternative representation of the smooth ambiguity preferences.
刘芳达 (加拿大滑铁卢大学)
题目:Insurance design with likelihood ratio uncertainty
摘要: The model uncertainty is of crucial importance when market participants are making risk management strategies. From a conservative consideration, decision makers seek the worst-case scenario among all conceivable models for a given risk measure. In this work, we use the likelihood ratio to quantify discrepancies between models, and study the optimal reinsurance strategy for an insurance company in the worst-case scenario. We establish a link between the insurer’s optimal strategies in scenarios with and without the model uncertainty. If the insurer’s optimal reinsurance is known under the reference model, applying our method, our approach facilitates the direct determination of the insurer’s optimal strategy, accounting for the likelihood ratio model uncertainty.
王若度 (加拿大滑铁卢大学)
题目:An unexpected stochastic dominance: Pareto distributions, catastrophes, and risk exchange
摘要: We show the perhaps surprising inequality that the weighted average of independent and identically distributed super-Pareto random variables, possibly caused by triggering events, is larger than one such random variable in the sense of first-order stochastic dominance. The class of super-Pareto distributions is extremely heavy-tailed and it includes the class of infinite-mean Pareto distributions. We discuss several implications of this result via an equilibrium analysis in a risk exchange market. First, diversification of super-Pareto losses increases portfolio risk, and thus a diversification penalty exists. Second, agents with super-Pareto losses will not share risks in a market equilibrium. Third, transferring losses from agents bearing super-Pareto losses to external parties without any losses may arrive at an equilibrium which benefits every party involved. The empirical studies show that our new inequality can be observed empirically for real datasets that fit well with extremely heavy tails.
韩霞 (南开大学)
题目:Choquet regularization for reinforcement learning and its application
摘要: We propose Choquet regularizers to measure and manage the level of exploration for reinforcement learning (RL), and reformulate the continuous-time entropy-regularized RL problem of Wang et al. (2020, JMLR, 21(198), 1-34) in which we replace the differential entropy used for regularization with a Choquet regularizer. Under the linear-quadratic setting, we derive explicit optimal distributions for several specific Choquet regularizers, and conversely identify the Choquet regularizers that generate a number of broadly used exploratory samplers such as -greedy, exponential, uniform and Gaussian. We further design a RL algorithm to solve the exploratory mean-variance problem and test our RL algorithm via simulation.
张瑞勋 (北京大学)
题目:Spectral Volume Models: Universal High-Frequency Periodicities in Intraday Trading Activities
摘要: We develop spectral volume models to systematically estimate, explain, and exploit the high-frequency periodicity in intraday trading activities using Fourier analysis. The framework consistently recovers periodicities at specific frequencies in three steps, despite their low signal-to-noise ratios. This reveals important and universal high-frequency periodicities across 2,573 stocks in the United States (US) and Chinese markets over three full years. The dominant frequencies explain a significant fraction of the total variance of intraday volumes. Through three different perspectives, we provide evidence that this phenomenon likely reflects the behaviors of trading algorithms with repeated and regular trading instructions. Finally, we demonstrate the practical value of uncovering these high-frequency periodicities in improving intraday volume predictions and in understanding return risk factors. Long-short portfolios constructed based on a periodicity factor yield monthly alphas of up to 0.9% in the US and 5% in China.
刘敬真 (中央财经大学)
题目:Optimal investment-consumption-insurance for Households with habit formation
摘要: Household asset allocation is an essential topic in recent years. The paper introduces habit formation into the optimal investment, consumption and life insurance decisions of a two-person family over [0,T ] period. We transform the original optimization problem into a two-stage problem. For the two stages respectively, the Hamilton-Jacob-Bellman equations satisfied by the value function are solved with the dynamic programming method. The analytic solutions of the problem is obtained under the case of CRRA utility function. Moreover, we use the Chinese data to conduct a numerical simulation and analyze the influence of habit formation on the optimal strategies. It is shown that when habit formation is considered, the optimal consumption expenditure increases with time. The portion of excess consumption decreases with the increase of habit formation. The habit formation squeezes the life insurance expenditure and raises the household saving rate, resulting in a decrease in risky asset investment and life insurance expenditure. We also found the less dependent a household is on the past consumption, the smoother the household consumption.
王秋淇 (美国佐治亚州立大学)
题目:Distributionally Robust Optimization of Distortion Riskmetrics and Optimal Insurance
摘要: We study optimization problems of distortion riskmetrics under distributional uncertainty. We first show a unifying result that allows us to convert an optimization of a non-convex distortion riskmetric with distributional uncertainty to a convex one, leading to great tractability. A sufficient condition to the unifying equivalence result is the novel notion of closedness under concentration. Adopting the concept of concentration, we apply our theory to an optimal insurance problem with a deductible contract. Within this framework, our major result shows sufficient conditions for the worst-case non-convex distortion risk measure to be equal to its convex counterpart. Under a weaker condition, we show the equivalence also holds when the outer problem on the deductible is considered. Distributionally robust optimization problems on Value-at-Risk, Expected Shortfall, and other common distortion risk measures will be presented as special examples of our results.
臧鑫 (北京交通大学)
题目:Random distortion risk measures
摘要: This paper presents a random risk measure, named as the random distortion risk measure. The random distortion risk measure is a generalization of the traditional deterministic distortion risk measure by randomizing the deterministic distortion function and the risk distribution respectively, where a stochastic distortion is introduced to randomize the distortion function, and a sub-sigma-algebra is introduced for illustrating the influence of the known information on the risk distribution. Some theoretical properties of the random distortion risk measure are provided, such as normalization, conditional positive homogeneity, conditional comonotonic additivity, monotonicity in stochastic dominance order, and continuity from below, and method for specifying the stochastic distortion and the sub-sigma-algebra is provided. Based on some stochastic axioms, the representation theorem of the random distortion risk measure is proved. For considering the randomization of a given deterministic distortion risk measure, some families of random distortion risk measures are introduced with the stochastic distortions constructed from Poisson process, Brownian motion and Dirichlet process respectively, and numerical analysis is carried out for showing the influence of the stochastic distortion and the risk distribution by focusing on the sample mean, variance, skewness, kurtosis, and the tail behavior of the random distortion risk measures.
Authors: Xin Zang, Fan Jiang, Chenxi Xia, Jingping Yang



